Fowler's Physics Applets
Fowler's Physics Applets
Michael Fowler - University of Virginia Physics
One-dimensional one-atom classical gas
This applet describes a single atom gas moving in one dimension. It accelerates or decelerates only through classical collisions with the moving piston on its container. This alone is enough to explain why the gas gets warm when it is compressed and cool when expanded.
Newton's Mountain
Newton's imaginary cannon is on a high mountain, and fires a cannonball above the atmosphere. Depending on speed,it could fall, circle the earth, or fly away depending on how hard it was fired, showing the motion of an cannonball near the Earth and the circling motion of the Moon around the Earth are different aspects of the same thing.
Rutherford Scattering
Rutherford scattering from a Thomson Atom and from a Nuclear Atom - Relevant Lecture in Physics 252 Modern Physics.
Watch alpha particles scatter from Thomson's model atom with charge spread evenly through all the atom's volume (as most people believed), and another model with all the charge concentrated in a small central nucleus.These were the two competing theories of atomic positive charge distribution in 1910. The startling experimental discovery of occasional large angle scattering proved there had to be a nucleus. This was the birth of nuclear physics.Nuclear Chain Reaction
This applet shows how a chain reaction develops. A nucleus in the middle emits a neutron in a random direction, if the neutron hits another nucleus it is absorbed, the hit nucleus emits two neutrons and dies.
A full chain reaction, involving essentially all the nuclei, is much more likely to occur with a bigger piece of material. Try it!
Einstein's Explanation of Brownian Motion
This applet shows how atomic velocities cause the Brownian Motion of a dust particle. In one panel a small ball jitters. In the next, we see that it jitters because many smaller balls bat it rapidly about. Here's the original Java version.
The Michelson-Morley Experiment
The classic attempt to detect the Earth's motion through the ether: light waves were thought to be oscillations in the ether, analogous to sound waves through air -- so should have slightly different velocities in different directions, with, against, or across the ether flowing past the Earth. The apparatus is a race course between light going up and down stream, and light going accross and back, which should be a little quicker, a detectable difference.
No ether was found -- this played a role in Einstein's creation of the theory of relativity.
Turn the turntable by dragging.
Time Dilation from a Lightclock
A clock bouncing light between two mirrors is animated, to show vividly why taking the speed of light the same in all inertial frames leads inevitably to time dilation.
Projectile Motion as Compound Motion
Here is Galileo's own diagram explaining that the parabolic path of a projectile, or a ball rolling off the edge of a table, can be regarded as compounded of horizontal motion at constant speed, plus vertical motion identical to that of a ball falling vertically. We've animated his drawing.
Projectile Motion
Shoot a cannon (or throw a ball!) to see how high and far the ball flies.> Here's the original Java applet.
Forces on a bit of a waving string
The waving motion of a string can be understood from Newton's laws if you think about a little bit of it, and the force on it at any instant as the imbalance of the tensions tugging at the two ends.
Visualizing a Sound Wave
A sound wave is waves of compression and rarefaction generated by a vibrating object. As the wave travels through the air, it gives the impression of carrying some air along, but actually air stays where it is, vibrating about its rest position. To see how this works, view the animation!
Wave Pulse Meets End of String
A wave pulse traveling down a uniform string under tension comes to the end of the string. A fixed end reflects it upside down, a free end reflects without inversion. This is explained by comparing with two approaching pulses on a string twice as long.
Wave Pulse Encounters Different Medium
A wave pulse traveling down a uniform string under tension comes to a join with a string of different density. The pulse is partially transmitted, partially reflected. The relative densities of the two strings can be set by the slider. Note the phase of the reflected wave as a function of relative string densities. The physics is the same for a wide variety of wave reflection phenomena.
Doppler Effect
Two-Slit Wave Interference Pattern
Synchronized waves emanating from two slits form a pattern with destructive interference in certain directions, where the waves are out of phase.
The slit separation and wavelength can be adjusted to explore how the pattern changes.
Building the Two Slit Pattern on a Screen
We move across the screen point by point to see how the two waves successively augment and cancel one another.
The slit separation and wavelength can be adjusted to explore how the pattern changes.
Carnot Cycle
Diffusion
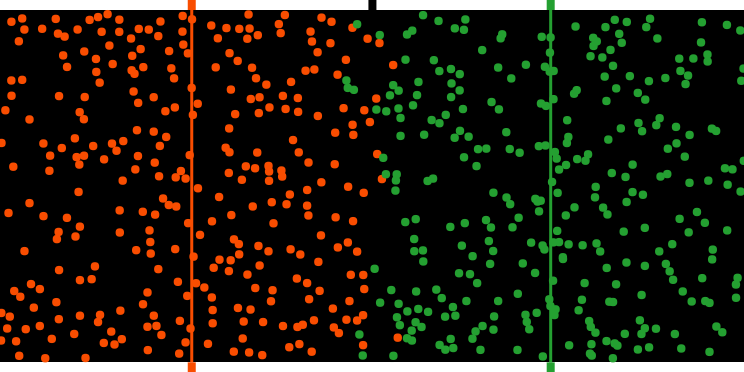
A box has red particles in the left hand half, green in the right. They all begin with the same speed but random direction. Watch as they mix! How long will it take? The vertical lines give the average positions of red and green particles.
Try different numbers of particles and different sizes.
Maxwell Molecular Speed Distribution
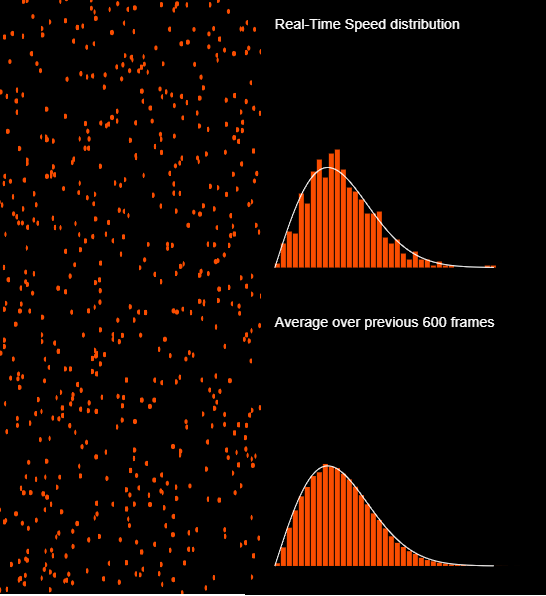
A dynamic realization: initially we give all molecules the same speed, collisions rapidly spread them out into Maxwell's predicted pattern.
The yellow curve is the theoretical prediction for Maxwell's distribution in two dimensions. (It begins linear, the three-dimensional distribution begins parabolic.)
Exponential Atmosphere: Dynamics + Gravity
Dynamics of Viscous Flow
A dynamic realization: initially we give all molecules the same speed, but a steady gravitational force pushes them down the pipe. On encountering the walls, they lose their horizontal (along the pipe) velocity. Vertically, they bounce off. The velocity down the pipe approaches a parabolic profile.
Newton's Picture of Acceleration in Circular Motion
A little Mathematics...
Kinetic Proof of Pythagoras' Theorem
Prove Pythagoras' theorem by moving the triangles around--in the original configuration, the total area inside the red square is equal to that of the four identical triangles plus the area of the central square on the hypotenuse (side = triangle's longest side). Rearrange to see that the total area inside the red square is also that of the four triangles plus the sum of the areas of squares on the other two sides.
The Platonic Solids
Hypocycloids
Cycloid
Simple Harmonic Motion is a Shadow of Circular Motion
Group Velocity and Phase Velocity
Change the group and phase velocities of interfering sine waves. Relevant Lecture for Physics 252 Modern Physics.
Some Astronomy Applets
Eclipse of the Moon
A short movie of the Moon entering the Earth's shadow, and how it appears from Earth. The ancient Greeks used this picture to estimate the distance to the Moon. Their estimate was within ten per cent of the correct answer.
The Inner Planets
A simple applet showing the relative orbital sizes and periods of the inner planets: Mercury, Venus, Earth and Mars.
The Outer Planets
A simple applet showing the relative orbital sizes and periods of the outer planets:Jupiter, Saturn, Uranus and Neptune.
Ptolemy's Model of the Orbital Motion of Venus and Mercury
This applet is a slightly simplified representation of Ptolemy's mode for the motion of the inner planets. From our modern perspective, the planets do not go in perfect circles around the Sun. If they did, our simplified Ptolemy model of uniform circular motion around an epicycle which is itself precessing uniformly would represent exactly the motion of the planet through the heavens. In fact, this is very close to the truth: the true orbits are ellipses, but very close to circular. Ptolemy's model had to be refined with small extra epicycles, or not quite uniform circular motion.
Here we just want to emphasize the main point: how this simple model can successfully account for the motion of the planets in the heavens to a very good approximation, but keeps the Earth itself at rest.
Point to notice: Venus and Mercury never get very far from the Sun in the sky. Think about how that works in the modern picture.Ptolemy's Model of the Orbits of Mars and Jupiter
An applet demonstrating that the motion of Mars through the heavens agrees exactly with the Copernican (modern) view of the Solar System and the ancient Earth-centered epicyclic model of Ptolemy.
It follows that observing the path of Mars through the stars, (or the path of any other planet, by similar model comparisons) cannot settle which model physically represents the real Solar System.
The Tusi Couple
In the 1260's, al Tusi, an astronomer in Iran, created an ingenious model to represent an observed small linear oscillatory component of planetary motion in terms of combined circular motions, thus extending Ptolemy's model. Here is his model .Observing Mars: Ptolemy or Copernicus?
An applet demonstrating that the motion of Mars through the heavens agrees exactly with the Copernican (modern) view of the Solar System and the ancient Earth-centered epicyclic model of Ptolemy.
It follows that observing the path of Mars through the stars, (or the path of any other planet, by similar model comparisons) cannot settle which model physically represents the real Solar System.
Platonic Solids: Kepler's idea for planetary orbital ratios
Kepler theorized that the planets moved on spherical surfaces separated by having Platonic solids just fit between neighboring orbits: see his model here.
Kepler's Laws
Planning a Trip to Mars
This applet plots the orbital motion of a spaceship launched from Earth at a given speed. Find the best orbit to Mars by launching at different speeds and at different times, to meet with Mars as it moves around its orbit.
Phases of Venus
This applet shows Venus' orbit from different perspectives to understand how the sunlit half of the planet exhibits phasess like the Moon.
General Planet: Central Forces ∝ Rn
This interactive applet helps explore the kinds of orbits generated by different central force laws: ellipses from the inverse square law of our Solar System, how a slight deviation from inverse square causes orbital precession, the clue that helped lead Einstein to general relativity, and a wide variety of other orbits.
Text is available under the Creative Commons Attribution/Share-Alike License.