previous  index  next 

33  Magnetic Materials: Relating B to H

    Michael Fowler, UVa

The Constitutive Relation

There’s one piece missing in the discussion of magnetostatics so far. How strong is the magnetic response to an external field? The electrostatic parallel was how much polarization an electric field generated, the so-called constitutive relation was $\overrightarrow{D}=\epsilon \overrightarrow{E}.$ For magnetism, the constitutive relation is $\overrightarrow{B}=\mu \overrightarrow{H},\mu$ being termed the magnetic permeability. Looking at actual materials, this simple linear relation works well for diamagnets and paramagnets, but things are much more complicated for ferromagnetic materials, as discussed below.

Diamagnets and Paramagnets

Just as a dielectric in an external electric field becomes polarized, equivalent to getting a finite density of electric dipoles, applying an external magnetic field to a magnetic material can induce magnetization, which$—$macroscopically$—$is equivalent to a density of magnetic dipoles. But just what are these dipoles? We know they’re not pairs of monopoles.

One contributor is molecular current loops, as envisaged by Ampere. So let’s pretend some electron circling a nucleus is replaced by a current in a wire loop. It of course has a magnetic moment. Now suppose we turn on an external magnetic field. What happens? Well, as you should remember from undergraduate physics, there’s Lenz’ law: the current will change in such a way as to oppose the change in field. Of course, the atom is really a quantum mechanical object, but a more sophisticated analysis reaches the same conclusion$—$the atomic orbitals will rearrange to oppose the change in field. This means the orbits create their own new magnetic field, opposing the one imposed.  This is called diamagnetism. The susceptibility is of order 10^-4 to 10^-5. If the atom can move, it will move towards weaker field.  Although the effect is very small, it's readily observable with a strong enough field: water, for example, is diamagnetic, and there's a famous movie of a frog suspended in a magnetic field thanks to being mainly composed of water.

Superconductors are the one exception to the general rule that diamagnetism is weak.  In a piece of superconducting material, surface currents will be generated to exclude the magnetic field entirely from the material, provided the field is below a certain strength. That is, a superconductor is a perfect diamagnet.

So why isn’t everything diamagnetic? In fact, everything made of atoms is, but there’s more. The electron itself has a magnetic moment antiparallel to its spin. But in water, for example, the electrons are in pairs of opposite spins, so the spin magnetic moments cancel. But for an atom or molecule with an odd number of electrons, the total electron spin is necessarily nonzero, and an electron acts as a point dipole, not a current loop, and so will tend to align with a magnetic field.   Such atoms are called paramagnetic.

Both diamagnetics and paramagnets respond weakly to external fields, and revert to their previous state when the field is removed. The important magnetic materials are the ferromagnets.

Ferromagnets

Ferromagnets, like iron, cobalt, nickel and many alloys including these metals, are dramatically different: they have a huge response to an applied magnetic field, and stay magnetized, at least to some extent, when the field is turned off. So what’s the big difference? Part of the story is that they have lined up spins within each atom, but the most important factor is that there are very strong quantum-mechanical forces between neighboring atoms tending to align them. These forces are hundreds of times stronger than the magnetic dipole-dipole interaction between the same atoms.

Beginning inside individual atoms, those with inner electron orbital shells close to half filled tend to have the spin wave function as symmetric as possible, meaning maximum possible alignment of the individual spins. The traditional explanation of this (Hund’s rule) was that with high spin symmetry, to get the required overall fermion wave function antisymmetry, the spatial wave function would need to be antisymmetric, so electrons couldn’t get too close, thereby lowering Coulomb energy. More recent wave function calculations suggest that having only one spin state occupied in a single spatial orbital means the electron gets closer to the nucleus, so is more tightly bound. We don’t need to pursue these chemical details here, all we need is that the strong magnetism of transition metals and rare earths is mostly from aligned electron spins in partly-filled inner shells, not so much from orbital motions$—$in fact, the half-filled shell has zero total orbital angular momentum, and therefore zero orbital magnetic moment).

Aligning Spins and Forming Domains

 As well as the intra-atomic spin alignment forces, in ferromagnets there are strong quantum interactions from overlapping wave functions between neighboring atoms and groups of atoms tending to align atomic spins (parallel or antiparallel, depending on the atoms involved$—$this can get very complicated.)  In fact at room temperature almost all the atoms in a piece of iron have spins parallel to their neighbors. So why isn’t every piece of iron almost fully magnetized? It’s because if all the spins in the piece point the same way, they create a very strong dipole-like external magnetic field, which takes a lot of energy, as we’ll discuss shortly.

So, to go to a lower energy state, the iron divides into domains, in each of which the spins are aligned with each other, but the domains have different spin orientation, greatly reducing the macroscopic field, and so going to a lower overall energy.   A simple example is an iron whisker (shown here).

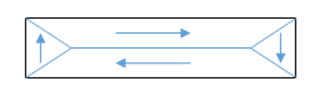
Of course, the boundaries between domains cost some energy to create (including elastic stress energy from anisotropies in fitting differently oriented domains together), but this is evidently less than the energy gained by drastically reducing the overall magnetic field.

The domains in the iron whisker can be observed as an external magnetic field is turned on. This of course changes the energy balance$—$domains aligned with the field now have lower energy. What happens is that the aligned domains grow at the expense of the others. The boundary walls move around$—$but not completely freely. No crystal is perfect, there are dislocations of the lattice structure, and impurities, not to mention the surfaces and edges of the crystal. Experimentally, it is found that the moving domain wall can get pinned by these irregularities, and extra energy, like an increased external field, is necessary to keep it moving.

Soft and Hard Ferromagnets; Hysteresis

Ferromagnetic materials are often referred to as soft and hard, magnetically speaking. A soft material readily aligns to an external field. This is what you want in an electromagnet, which is basically a solenoid with a ferromagnetic core. Hard materials need strong fields to become magnetized, but then retain their magnetization very well$—$these are needed for permanent magnets. The essential difference between the two materials is the ease with which domain walls between areas of different magnetization direction can move in response to an external field, and this is determined by the density of “pinning centers” where the moving wall gets stuck. These can be crystalline imperfections, such as vacancies or dislocations of the lattice, or impurities, which may have been deliberately placed to harden the magnetic properties. 

For ferromagnets, the relationship is nonlinear: see the diagram.  The field $H_{\text{ext}}$ is provided by free currents in external coils. For annealed iron, an external field of a few gauss can magnetize the iron so that it has a field of one tesla or so. Increasing the external field has little effect$—$the iron atoms are all aligned at this point. Magnetism disappears above a certain temperature, which depends on the material. For iron, it is close to 1000K.

Experimentally, the linear relation $\overrightarrow{B}=\mu {\overrightarrow{H}}_{\text{ext}}$ fails to describe the response of an unmagnetized piece of ferromagnetic material to an increasing external magnetic field, except at the very beginning, where there is linear increase with values of $\mu$ varying from 100 to 1,000,000.  After that initial linear increase, $M$ increases rapidly, finally saturating when all the molecular moments are lined up with the field. 

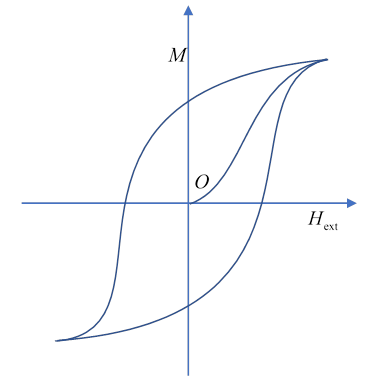
On cycling the external field as shown, the magnetization cycles, but with a lag, called hysteresis. This is effectively internal friction between blocks of magnetized spins attempting to realign as the external field changes. We can also see that simply removing the external field will leave a permanent magnetization (which can be degraded by temperature, vibration, or another magnetic field). 

To see the huge difference between soft and hard ferromagnets, compare the magnetization curve for very soft iron with that for alnico, a material for making permanent magnets. (Feynman uses the old units: all measured in gauss.)

To see more recent data, check this: the silicon steel used in motors, etc., is laminated, so anisotropic. The area enclosed in the hysteresis curve represents energy loss per cycle (from resistance to domain wall movement) so is extremely important from a practical point of view. The hysteresis loop tops out at saturation, this limits the field strength possible. Tnere are other energy losses from eddy currents (which we discuss later), they are comparable in magnitude to the hysteresis losses.

We’ve plotted $M$ vertically, not $B$ as Jackson does, for ferromagnetic materials the difference is negligible$—$except for units. The field $B$ has dimensions defined by the force on a moving charged particle, $\overrightarrow{F}=e\overrightarrow{v}\times \overrightarrow{B},$ from which $\left[B\right]=M{I}^{-1}{T}^{-2}=M{Q}^{-1}{T}^{-1}$ with $I,Q$ denoting current and charge.  The SI unit is the tesla, aka volt-seconds per square meter.  The old unit was the gauss, one tesla is 10,000 gauss.  The units for magnetization can be figured out from the current equivalence, ${\overrightarrow{j}}_M=\overrightarrow{\nabla }\times \overrightarrow{M},$ the current density is amps per square meter, so the units of magnetization $\left[M\right]=I{L}^{-1}=\left[H\right],$   and $\left[\mu \right]=\left[{\mu }_0\right]=ML{T}^{-2}{I}^{-2},$ sometimes written as volt-seconds/ampere-meters or  henry/meter. (The henry is a unit of inductance, we’ll get to it soon.)

Recommended Reading

This is not a course on Condensed Matter, so we can spend no more time on the fascinating subject of magnetic materials. We have only skimmed the surface.  I strongly recommend the very readable Feynman’s notes on magnetism!   You can also check in Wikipedia more recent progress on some of the unresolved mysteries Feynman discusses.

previous  index  next